Dating radioactive isotopes
Contents:
Because isotopes differ in mass , their relative abundance can be determined if the masses are separated in a mass spectrometer see below Use of mass spectrometers. Radioactive decay can be observed in the laboratory by either of two means: The particles given off during the decay process are part of a profound fundamental change in the nucleus. To compensate for the loss of mass and energy , the radioactive atom undergoes internal transformation and in most cases simply becomes an atom of a different chemical element.
In terms of the numbers of atoms present, it is as if apples changed spontaneously into oranges at a fixed and known rate. In this analogy , the apples would represent radioactive, or parent, atoms, while the oranges would represent the atoms formed, the so-called daughters. Pursuing this analogy further, one would expect that a new basket of apples would have no oranges but that an older one would have many.
In fact, one would expect that the ratio of oranges to apples would change in a very specific way over the time elapsed, since the process continues until all the apples are converted. In geochronology the situation is identical. A particular rock or mineral that contains a radioactive isotope or radioisotope is analyzed to determine the number of parent and daughter isotopes present, whereby the time since that mineral or rock formed is calculated. Of course, one must select geologic materials that contain elements with long half-lives —i.
The age calculated is only as good as the existing knowledge of the decay rate and is valid only if this rate is constant over the time that elapsed. Fortunately for geochronology, the study of radioactivity has been the subject of extensive theoretical and laboratory investigation by physicists for almost a century.
The results show that there is no known process that can alter the rate of radioactive decay. By way of explanation it can be noted that since the cause of the process lies deep within the atomic nucleus, external forces such as extreme heat and pressure have no effect. The same is true regarding gravitational, magnetic , and electric fields , as well as the chemical state in which the atom resides. In short, the process of radioactive decay is immutable under all known conditions. Although it is impossible to predict when a particular atom will change, given a sufficient number of atoms, the rate of their decay is found to be constant.
The situation is analogous to the death rate among human populations insured by an insurance company. Even though it is impossible to predict when a given policyholder will die, the company can count on paying off a certain number of beneficiaries every month. The recognition that the rate of decay of any radioactive parent atom is proportional to the number of atoms N of the parent remaining at any time gives rise to the following expression:.
Converting this proportion to an equation incorporates the additional observation that different radioisotopes have different disintegration rates even when the same number of atoms are observed undergoing decay. Solution of this equation by techniques of the calculus yields one form of the fundamental equation for radiometric age determination,.
Two alterations are generally made to equation 4 in order to obtain the form most useful for radiometric dating.
Navigation menu
In the first place, since the unknown term in radiometric dating is obviously t , it is desirable to rearrange equation 4 so that it is explicitly solved for t. Half-life is defined as the time period that must elapse in order to halve the initial number of radioactive atoms. The half-life and the decay constant are inversely proportional because rapidly decaying radioisotopes have a high decay constant but a short half-life. With t made explicit and half-life introduced, equation 4 is converted to the following form, in which the symbols have the same meaning:. Alternatively, because the number of daughter atoms is directly observed rather than N , which is the initial number of parent atoms present, another formulation may be more convenient.
Since the initial number of parent atoms present at time zero N 0 must be the sum of the parent atoms remaining N and the daughter atoms present D , one can write:. Substituting this in equation 6 gives. If one chooses to use P to designate the parent atom, the expression assumes its familiar form:. This pair of equations states rigorously what might be assumed from intuition , that minerals formed at successively longer times in the past would have progressively higher daughter-to-parent ratios.
This follows because, as each parent atom loses its identity with time, it reappears as a daughter atom. Equation 8 documents the simplicity of direct isotopic dating. The time of decay is proportional to the natural logarithm represented by ln of the ratio of D to P.
In short, one need only measure the ratio of the number of radioactive parent and daughter atoms present, and the time elapsed since the mineral or rock formed can be calculated, provided of course that the decay rate is known. Likewise, the conditions that must be met to make the calculated age precise and meaningful are in themselves simple:. The rock or mineral must have remained closed to the addition or escape of parent and daughter atoms since the time that the rock or mineral system formed. It must be possible to correct for other atoms identical to daughter atoms already present when the rock or mineral formed.
The measurement of the daughter-to-parent ratio must be accurate because uncertainty in this ratio contributes directly to uncertainty in the age. Different schemes have been developed to deal with the critical assumptions stated above. In uranium-lead dating , minerals virtually free of initial lead can be isolated and corrections made for the trivial amounts present.
In whole-rock isochron methods that make use of the rubidium- strontium or samarium - neodymium decay schemes, a series of rocks or minerals are chosen that can be assumed to have the same age and identical abundances of their initial isotopic ratios. The results are then tested for the internal consistency that can validate the assumptions.
In all cases, it is the obligation of the investigator making the determinations to include enough tests to indicate that the absolute age quoted is valid within the limits stated. In other words, it is the obligation of geochronologists to try to prove themselves wrong by including a series of cross-checks in their measurements before they publish a result.
Radiometric Dating: Methods, Uses & the Significance of Half-Life
Such checks include dating a series of ancient units with closely spaced but known relative ages and replicate analysis of different parts of the same rock body with samples collected at widely spaced localities. The importance of internal checks as well as interlaboratory comparisons becomes all the more apparent when one realizes that geochronology laboratories are limited in number. Because of the expensive equipment necessary and the combination of geologic, chemical, and laboratory skills required, geochronology is usually carried out by teams of experts.
Most geologists must rely on geochronologists for their results.
- Radiometric dating - Wikipedia?
- You must create an account to continue watching.
- Radiometric dating.
In turn, the geochronologist relies on the geologist for relative ages. In order for a radioactive parent-daughter pair to be useful for dating, many criteria must be met. This section examines these criteria and explores the ways in which the reliability of the ages measured can be assessed. Because geologic materials are diverse in their origin and chemical content and datable elements are unequally distributed, each method has its strengths and weaknesses.
Of these, only the radioisotopes with extremely long half-lives remain. It should be mentioned in passing that some of the radioisotopes present early in the history of the solar system and now completely extinct have been recorded in meteorites in the form of the elevated abundances of their daughter isotopes. Analysis of such meteorites makes it possible to estimate the time that elapsed between element creation and meteorite formation. Natural elements that are still radioactive today produce daughter products at a very slow rate; hence, it is easy to date very old minerals but difficult to obtain the age of those formed in the recent geologic past.
This follows from the fact that the amount of daughter isotopes present is so small that it is difficult to measure. The difficulty can be overcome to some degree by achieving lower background contamination, by improving instrument sensitivity, and by finding minerals with abundant parent isotopes. Geologic events of the not-too-distant past are more easily dated by using recently formed radioisotopes with short half-lives that produce more daughter products per unit time.
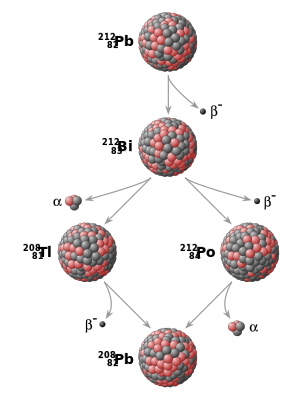
To understand this, one needs to know that though uranium U does indeed decay to lead Pb , it is not a one-step process. Absolute Time in Geology. Gillaspy has taught health science at University of Phoenix and Ashford University and has a degree from Palmer College of Chiropractic. Email Email is required. Deep time Geological history of Earth Geological time units. What is the Age of the Solar System?
The ions then travel through a magnetic field, which diverts them into different sampling sensors, known as " Faraday cups ", depending on their mass and level of ionization. On impact in the cups, the ions set up a very weak current that can be measured to determine the rate of impacts and the relative concentrations of different atoms in the beams.
Uranium—lead radiometric dating involves using uranium or uranium to date a substance's absolute age. This scheme has been refined to the point that the error margin in dates of rocks can be as low as less than two million years in two-and-a-half billion years. Uranium—lead dating is often performed on the mineral zircon ZrSiO 4 , though it can be used on other materials, such as baddeleyite , as well as monazite see: Zircon has a very high closure temperature, is resistant to mechanical weathering and is very chemically inert. Zircon also forms multiple crystal layers during metamorphic events, which each may record an isotopic age of the event.
- news about robert pattinson and kristen stewart dating?
- the best dating site in usa?
- i hate internet dating sites?
- Radiometric Dating?
One of its great advantages is that any sample provides two clocks, one based on uranium's decay to lead with a half-life of about million years, and one based on uranium's decay to lead with a half-life of about 4. This can be seen in the concordia diagram, where the samples plot along an errorchron straight line which intersects the concordia curve at the age of the sample. This involves the alpha decay of Sm to Nd with a half-life of 1. Accuracy levels of within twenty million years in ages of two-and-a-half billion years are achievable.
This involves electron capture or positron decay of potassium to argon Potassium has a half-life of 1. This is based on the beta decay of rubidium to strontium , with a half-life of 50 billion years.
This scheme is used to date old igneous and metamorphic rocks , and has also been used to date lunar samples. Closure temperatures are so high that they are not a concern. Rubidium-strontium dating is not as precise as the uranium-lead method, with errors of 30 to 50 million years for a 3-billion-year-old sample. A relatively short-range dating technique is based on the decay of uranium into thorium, a substance with a half-life of about 80, years.
It is accompanied by a sister process, in which uranium decays into protactinium, which has a half-life of 32, years. While uranium is water-soluble, thorium and protactinium are not, and so they are selectively precipitated into ocean-floor sediments , from which their ratios are measured. The scheme has a range of several hundred thousand years.
A related method is ionium—thorium dating , which measures the ratio of ionium thorium to thorium in ocean sediment. Radiocarbon dating is also simply called Carbon dating. Carbon is a radioactive isotope of carbon, with a half-life of 5, years, [25] [26] which is very short compared with the above isotopes and decays into nitrogen. Carbon, though, is continuously created through collisions of neutrons generated by cosmic rays with nitrogen in the upper atmosphere and thus remains at a near-constant level on Earth.
The carbon ends up as a trace component in atmospheric carbon dioxide CO 2. A carbon-based life form acquires carbon during its lifetime.